Standard Form of a Linear Equation Worksheet
Linear equations are fundamental in mathematics and find applications in various fields. Whether you're a student learning algebra or someone using equations in practical scenarios, understanding the standard form of a linear equation is crucial. This article will delve into the concept of standard form, its significance, conversion methods, practical applications, and more. Continue below >
Converting decimals to fractions
Converting decimals to fractions printable math worksheet
Converting fractions to decimals
Converting fractions to decimals printable math worksheet
Converting fractions to percents
Converting fractions to percents printable math worksheet
Converting percentage to decimals
Converting percentage to decimals printable math worksheet
Converting fractions to percents
Converting fractions to percents printable math worksheet
Complementary supplementary angles
Complementary supplementary angles printable math worksheet
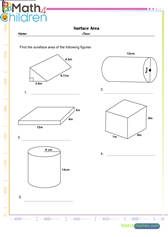
Surface area of complex figures
Surface area of complex figures printable math worksheet
Metric system converting scales
Metric system converting scales printable math worksheet
Ratio percent decimals fractions convertions
Ratio percent decimals fractions convertions printable math worksheet
Pre algebra multiplication addition
Pre algebra multiplication addition printable math worksheet
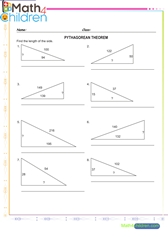
Triangle sides pythagorean theoream 6
Triangle sides pythagorean theoream 6 printable math worksheet
Triangle sides pythagorean theorem 1
Triangle sides pythagorean theorem 1 printable math worksheet
Triangle sides pythagorean theorem 2
Triangle sides pythagorean theorem 2 printable math worksheet
Triangle sides pythagorean theorem 3
Triangle sides pythagorean theorem 3 printable math worksheet
Triangle sides pythagorean theorem 4
Triangle sides pythagorean theorem 4 printable math worksheet
Triangle sides pythagorean theorem 5
Triangle sides pythagorean theorem 5 printable math worksheet
Triangle sides pythagorean theorem 7
Triangle sides pythagorean theorem 7 printable math worksheet
Introduction to Linear Equations
Linear equations represent relationships between variables that can be graphically represented as straight lines. They are expressed in the form of �=��+�, where � is the slope of the line, and � is the y-intercept. Solving linear equations helps in analyzing and predicting relationships in different situations.
Understanding Standard Form of a Linear Equation
The standard form of a linear equation is presented as ��+��=�, where �, �, and � are integers, and � is positive. This form offers a structured representation that simplifies various algebraic operations.
Benefits of Using Standard Form
- Uniform Representation: Standard form provides a consistent structure for linear equations, making it easier to compare and manipulate them.
- Ease of Graphing: Converting equations to standard form allows for straightforward graphing, aiding visualization and analysis.
- Compatibility: Many mathematical software and applications prefer equations in standard form, facilitating computational tasks.
How to Convert Linear Equations to Standard Form
Converting equations to standard form involves rearranging terms to meet the ��+��=� format. Here's a step-by-step guide:
- Remove Parentheses: Distribute any terms inside parentheses to eliminate them.
- Combine Like Terms: Group similar terms together.
- Isolate Variables: Move all variable terms to one side of the equation and constants to the other.
- Normalize Coefficients: Ensure that � is positive and all coefficients are integers.
Practice Problems with Solutions
To reinforce understanding, let's solve a few practice problems:
-
Given �=3�−2, convert the equation to standard form.
- Solution: −3�+�=−2
-
Convert 2�−5�=10 to standard form.
- Solution: 2�−5�=10
Applications of Standard Form in Real Life
Standard form equations find applications in various fields such as economics, physics, and engineering. They help model relationships in scenarios like cost analysis, motion calculations, and electrical circuit design.
Advantages and Disadvantages of Standard Form
Advantages:
- Provides a uniform representation.
- Facilitates easy comparison and manipulation.
- Compatible with mathematical software.
Disadvantages:
- Conversion process may be time-consuming.
- Requires careful handling of coefficients and constants.
Tips for Solving Standard Form Equations Efficiently
- Maintain Accuracy: Double-check all steps while converting equations to standard form.
- Practice Regularly: Familiarity with the conversion process enhances efficiency.
- Seek Assistance: Don't hesitate to ask for help when encountering challenging problems.
Common Mistakes to Avoid
- Neglecting to distribute terms inside parentheses.
- Forgetting to isolate variables properly.
- Ignoring the requirement for � to be positive.
Frequently Asked Questions
-
What is a linear equation?
- A linear equation represents a relationship between two variables that can be graphically depicted as a straight line.
-
Why is standard form important?
- Standard form offers a structured representation that simplifies comparison, manipulation, and computational tasks involving linear equations.
-
How do you convert an equation to standard form?
- The conversion involves rearranging terms to meet the ��+��=� format, ensuring � is positive and all coefficients are integers.
-
Can standard form be used for all linear equations?
- Yes, standard form can represent any linear equation, but the conversion process may vary depending on the given equation.
-
What are some real-life examples of standard form equations?
- Examples include cost functions, profit equations, and equations modeling physical phenomena like motion or heat transfer.
In conclusion, mastering the standard form of linear equations is essential for anyone dealing with mathematical concepts. Its structured representation simplifies analysis and facilitates applications in diverse fields.